Space-time tells matter how to move; matter tells space-time how to curve - John Wheeler
Greetings, fellow Bohron

The equivalence principle not only unified acceleration and gravity as one thing but also forced us to ponder upon the true nature of gravity. At Kaiser Wilhelm Institue of Physics, Einstein could continue his research without any distractions and financial worries. He started where Newton had left two centuries earlier.
Special vs General Relativity
Back in 1905, Einstein wasn’t able to visualize the relation between gravity and acceleration. In Special Relativity, we consider spacetime as flat and that it obeys Euclidean Geometry, the simple maths which we all have learned in high school.
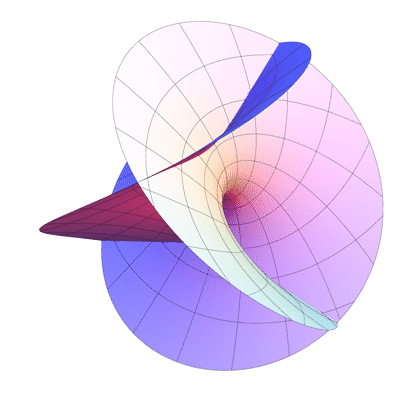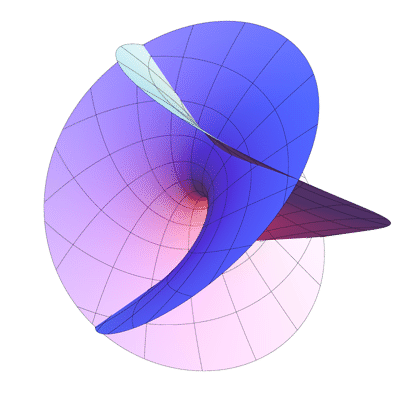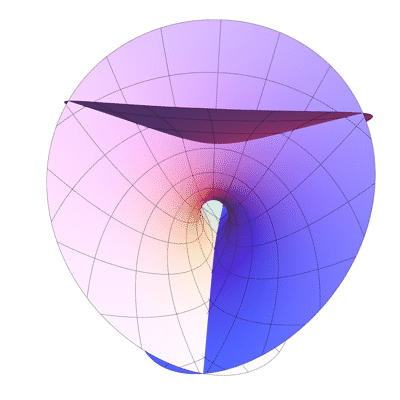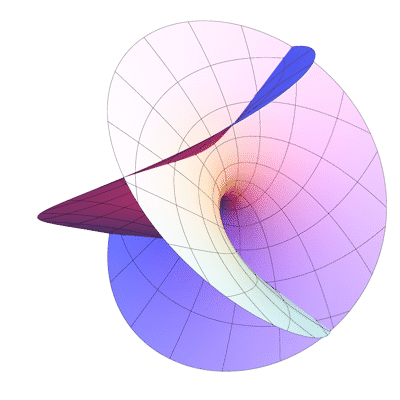
A decade later, when he stumbled upon the fact that acceleration and gravity are equivalent, he realized that spacetime is in fact curved and that it obeys Riemannian Geometry in which some of the basic and obvious looking mathematical notions don’t hold true.
Special Relativity applies only to non-accelerated reference frames. Einstein’s next challenge was to extend this theory to non-inertial frames as well.
Einstein’s Picture of Gravity
General Relativity describes gravity as a result of the warping of the spacetime fabric due to the distribution of mass and energy in it.

Consider a heavy spherical mass lying at the centre of a trampoline. The surface of the trampoline is curved inwards due to the stress it causes on its fabric. Greater is the mass of the body, more is the bending produced.
Now take lighter sphere and drop it on the trampoline. It also warps the fabric, but it is very small as compared to that of the heavier mass. The lighter sphere is attracted towards the heavier sphere.

According to Einstein, this is exactly how gravity works.
Geodesics
A straight line is the shortest distance between two points on a flat surface. What about the shortest distance between two points in a curved space when you are restricted to its surface?

Think of walking from New York to London. Although you may think you’ve been following a straight line path all the way, however, since Earth’s surface is curved, you actually trace out a curve.
A Geodesic is the shortest path between two points on a curved surface. It is an equivalent of a straight line in the Riemannian Manifold.
Bodies always follow the straight lines in 4D spacetime but they appear to us to move along curved paths in 3D space. Geodesics play an important role since they help us predict the path a body will follow in the curved spacetime.
Grossman Comes to the Rescue

The mathematics of curved spaces is too perplexing, and even Einstein understood that he needed a helping hand at the moment. He contacted his old friend Marcel Grossman, a Swiss mathematician who specialized in non-euclidean geometry.
Einstein field equations in their simplest form describe how the geometry of spacetime is affected locally by the presence of mass and energy. When fully opened up, they form a set of ten non-linear differential equations which need to be solved fully to make predictions about the future events.

Grossman assisted Einstein with tensor calculus and differential calculus, to construct the most beautiful framework ever build to describe Gravitation. They together wrote a paper: Outline of a Generalized Theory of Relativity and of a Theory of Gravitation, one of the two fundamental papers which established Einstein's theory of gravity.





Great explanation ☺️👌👌enjoyable and. Knowledgable
Really very interesting😇 thanks for such a great explanation 👍🏻